Entangled-Light-Emitting Diode

​

Research Links

• Entangled-Light-Emitting Diode
• Entanglement explanation

Conclusions

• Toshiba has a quantum information group
• Likely that a standard LASER's photons are disentangled if they are ever entangled by a "which path" scenario as mentioned below.

Excerpted from Toshiba page:

For some important applications, quantum computers have potentially massive processing power, due to the way data is encoded upon quantum bits (qubits). One of the resources required to operate an optical quantum computer, is entangled light. At Toshiba, our research on entangled light sources has resulted in many important achievements. These include realisation of the first semiconductor source of triggered entangled photons, creation of time-evolving entangled light states, and recently the first electrically driven source of entangled light.

Entangled light possesses the unusual feature that its constituent particles (photons) have inter-related properties, in this case polarisation. Measurement of one photon affects the polarisation of the other, even if they are separated by huge distances. This curious phenomenon was famously declared by Einstein to be “spukhafte Fernwirkung” or “spooky action at a distance”. These properties of entangled light derive from the fact that according to quantum mechanics, the photon pair exist in a superposition state, and the polarisation of the pair is uncertain until measurement of one photon.

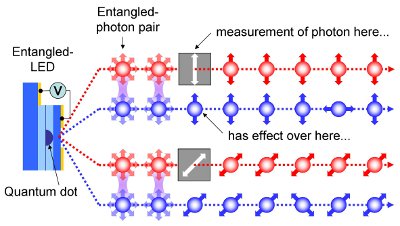
We create photon pairs using nanometer-scale regions of semiconductor known as quantum dots. Their small size means quantum dots can capture a maximum of two negative and positive charges (electrons and holes respectively). The electrons and holes recombine to emit a pair of photons.

However, photon pairs emitted by conventional quantum dots are not entangled, as the energies of the emitted photons are polarisation dependent. This means the polarisation of a photon can be determined by measurement of it's energy, providing the dreaded ‘which-path‘ information that is well know to destroy entanglement. We have solved this problem by pioneering a technique to optimise the size and shape of the quantum dot so that the energies of the emitted photons are equal, and entangled light can be emitted. This led to realisation of the first semiconductor source of triggered entangled photon pairs, which we achieved by driving a single quantum dot with a laser.

We have subsequently made many advances in the performance and operation of the device, which include enhanced resolution quantum interferometry, creation of time-evolving entangled states, and improvement of the fidelity, or purity, of the entangled light to 91%. However, entangled light produced previously by us and others requires a laser beam as a power source. For applications such as optical quantum computing that require many entangled photons, the practical advantages of creating entangled light by electrical current are very significant. In collaboration with the University of Cambridge, we now report in the journal Nature, realisation of the first electrically driven source of entangled photons.

Our device is based on a conventional light-emitting-diode (LED) structure, but additionally contains a specially optimised quantum dot. A voltage applied to the LED causes a current to flow, and the quantum dot captures the charge required to emit a pair of photon. In addition, the thickness of the semiconductor material surrounding the quantum dot was optimised to regulate the rate charge is transferred to the dot. Without this feature, entanglement is destroyed by extra charge. We demonstrate that the device works well in both d.c. and a.c. mode, with fidelities up to 82%.

An additional fundamental advantage of the entangled LED is that it has the potential to operate on demand, supplying one entangled pair nearly every cycle. When combined with the practical advantage offered by electrical excitation, the entangled LED will allow simultaneous operation of many entangled light sources on a single chip, opening the path to ultra-powerful semiconductor processors based on quantum computation.

Further Reading

 

 

 

 

 

	Type	Title and author(s)	Source
1	Technical	An entangled-light-emitting diode  by C L Salter et al.	Nature 465, 594–597 (2010)
2	Technical	Bell-inequality violation with a triggered photon-pair source  by R J Young et al.	Physical Review Letters 102, 030406 (2009)
3	Technical	Evolution of entanglement between distinguishable light states  by R M Stevenson et al.	Physical Review Letters 101, 170501 (2008)
4	Technical	Biphoton interference with a quantum dot entangled light source  by R M Stevenson et al.	Optics Express 15, 6507 (2007) [open access]
5	Technical	A semiconductor source of triggered entangled photon pairs  by R M Stevenson et al.	Nature 439, 179–182 (2006)
6	Technical	Inversion of exciton level splitting in quantum dots  by R J Young et al.	Physical Review B 72, 113305 (2005)